

On the later topics of integration techniques andĪpplications, polar coordinates, improper integrals, and infinite series. With 18.01A, a six-week class offering a very rapid review of one-variable calculus, focusing
#CALCULUS MATH FULL#
This sequence, intended for students who have had a full year of high school calculus, begins 18.0xA 18.01A-18.02A Calculus I and IIġ8.01A is given during the first six weeks of the Fall semester.ġ8.02A is begun during the second half of the Fall semester and completed during IAP or Spring On geometry, vector fields, and linear algebra. 18.022 Calculus IIĬovers the material of 18.02 with a more mathematical focus. Fall 18.02 AlternativeĪnother flavor of 18.02 is offered in the Fall semester. Integrals are introduced along with their application to concepts of work and flux, and studiedīy means of the theorems of Green, Gauss, and Stokes. Introduced to describe physical notions such as velocity and force fields. Vectors, and vector-valued functions, and vector fields are Partial differentiation and multiple integrationĪre studied with applications. The prerequisites for 18.01 are high school algebra and trigonometry any MITĬovers vector and multi-variable calculus.
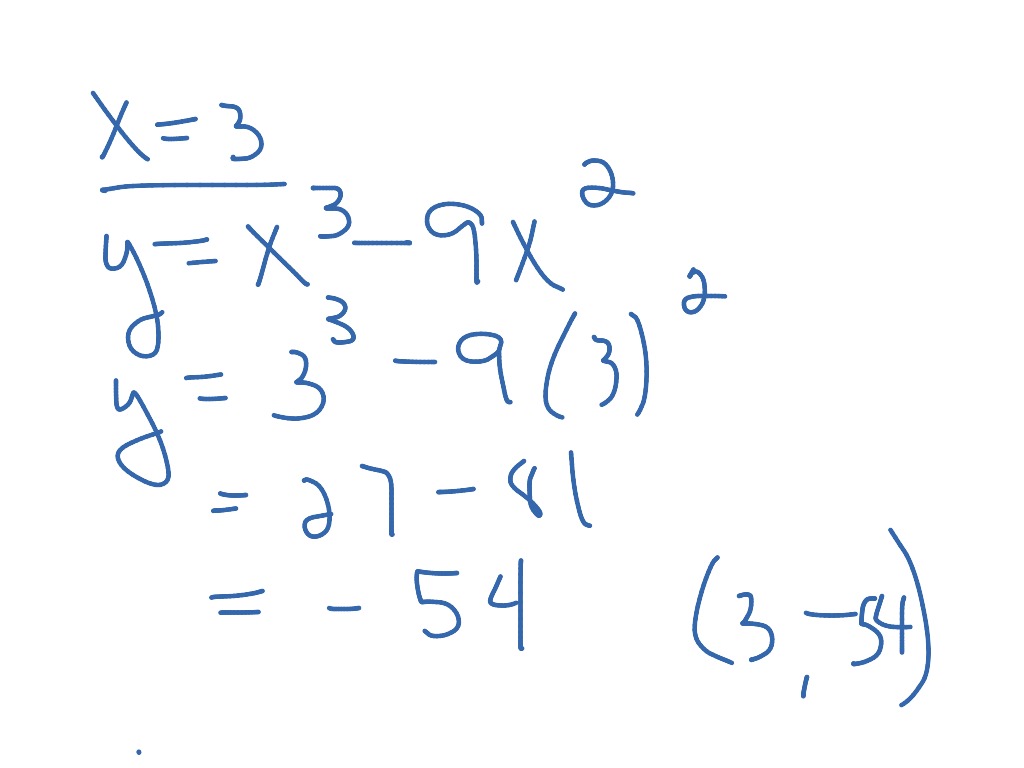
18.01 Calculus IĬovers differentiation and integration of functions of one variable, with some basicĪpplications. Some theorems are proved carefully while others are justified informally. Both subjects are offered in both fall and spring. The basic 18.01 - 18.02 calculus sequence is a traditional treatment adapted for MIT Any form of 18.01 credit fulfills the prerequisite for all forms of 18.02.

18.02 can be fulfilled by passing an Advanced Standing Exam or by transfer credit.Įach of 18.01 and 18.02 is offered in several forms, with different course numbers. The 18.01 requirement can also be fulfilled through suitable scores on tests such as Advanced Placement exams or by passing Advanced Standing Exams or by transfer credit. The Mathematics GIR consists of 18.01 and 18.02 or equivalent courses. The two-subject calculus General Institute Requirement (GIR) is designed to introduce the fundamental concepts of the calculus and provide the mathematical foundations on which subjects across MIT build. Mathematics is the common language of science and engineering, and calculus is a part of mathematics that is essential for understanding and describing many aspects of the physical world. Calculus Calculus - 2 Subjects, 12 Units Each


 0 kommentar(er)
0 kommentar(er)
